Treasure Island ★★![[New!]](../images/new.gif)

Robert has found a piece of parchment that describes where the treasure of famous pirate Redbeard is buried.
The text reads:
Travel to the wooden gallows on the island Vlieland.
From the gallows one can see the only two trees on the island, an elm and a beech.
Walk from the gallows to the elm and count the number of steps.
At the elm, turn 90 degrees clockwise and walk the same number of steps. Mark the location.
Go back to the gallows and walk from the gallows to the beech again counting the number of steps.
At the beech, turn 90 degrees counterclockwise and again walk the same number of steps. Mark the location.
The treasure is buried in the middle between the two marked locations.
Robert travels to the island Vlieland.
There he quickly finds the two trees, but the gallows apparently is gone, because it is nowhere to be found.

The question:
How can Robert find the treasure, without needing to plough up the whole island?
Cards with Crosses ★★

You have a stack of 23 cards.
Each card has an image of a cross on one side and an image of a circle on the other side.
You know that 14 cards in the stack are lying with the cross up, and therefore 9 cards are with the circle up.
However, you do not know in which order the cards are.
Moreover, you are in a completely dark room, so you cannot see anything.

The question:
How can you, without seeing anything, divide the stack of cards in two smaller stacks,
in such a way that both stacks have the same number of cards with a cross up?
Animals in Action ★★★

Vicious poachers caught four animals in the Great Animal Forest and put them into four separate cages.
The four animals are:
- the lion, that always tells the truth;
- the jackal, that always lies;
- the parrot, that always repeats the last given answer (if he is the first one, he randomly says "yes" or "no");
- the giraffe, that is so slow that he always truthfully answers the previous question (if he is the first one, he also randomly says "yes" or "no").
The other animals want to free them and send the small, smart hedgehog on the way to size up the situation.
In the middle of the night, the hedgehog creeps into the camp of the poachers and tries to figure out which animal sits in which cage.
One by one, he asks them "are you the jackal?"
After hearing the four answers, the hedgehog only knows in which cage the giraffe sits.
Then the hedgehog asks them in the same order "are you the giraffe?"
After hearing the four answers, the hedgehog also knows in which cage the jackal sits.
Because the hedgehog then still does not know in which cages all animals sit, he asks the first animal "are you the parrot?"
The answer is "yes", and now the hedgehog knows exactly which animal sits in which cage.
The hedgehog returns to the other animals and together they free the captured animals.

The question:
Which animal was in which cage?
Eureka! ★★★
There is a story that Archimedes, the Greek mathematician,
was asked to find out if the new golden crown of the king was made of pure gold,
while keeping the crown intact.
Sitting in a public bath and thinking about it,
Archimedes noticed the displacement of the water caused by sinking his body lower into the water.
He suddenly realized that he had found the solution: if the crown was made of pure gold,
it should displace the same volume of water as a bar of pure gold with an equal weight.
Excited, he jumped out of the bath and ran home shouting "Eureka!" ("I've found it!"),
forgetting that he was still naked...
We do not know if the story is true. However, we do know that Archimedes discovered the first law of hydrostatics:
when a body is immersed in a fluid,
it experiences an upward buoyant force, which is equal to the weight of the fluid displaced by the immersed part of the body.
Can you solve the following questions and have your "Eureka!" moments, using this famous law?
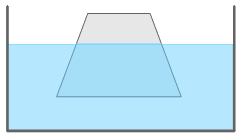
In an aquarium filled with water, a block of ice floats.
We mark the current water level.

The question:
When the ice has molten completely, will the water level be higher, lower, or still the same?

Another question:
In an aquarium filled with water, a block of ice block floats, with a bar of gold frozen in it.
We mark the current water level.
When the ice has molten completely and the bar of gold has sunk to the bottom of the aquarium,
will the water level be higher, lower, or still the same?

Yet another question:
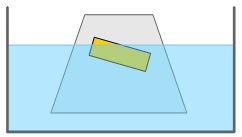
In an aquarium filled with water, a block of wood floats.
On top of the block of wood, a brick has been glued.
We mark the current water level.
If the block of wood is turned around (so that the brick hangs under it), will the water level rise, fall, or stay the same?
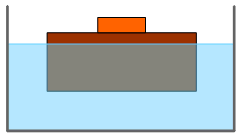

The fourth question:
We have a pair of scales, with a block of lead on the left scale, and a block of wood on the right scale.
Both blocks have the same weight, so the scales are in balance.
We take the scales with the blocks and immerse them in an aquarium filled with water.
Will the scales stay in balance, will they turn left, or will they turn right?


The fifth question:
In an aquarium filled with water, a block of wood floats.
On top of the block of wood, there lies a bar of gold.
We mark the current water level.
If the bar of gold falls into the water and sinks to the bottom of the aquarium, will the water level rise, fall, or stay the same?
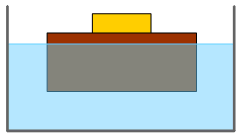

The sixth question:
In an aquarium filled with water, a sponge floats.
While the sponge slowly absorbs water (but stays floating), will the water level rise, fall, or stay the same?
The Bridge ★★★
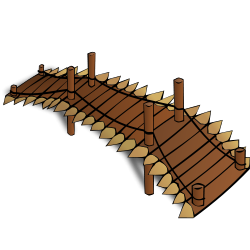
Four men want to cross a bridge. They all begin on the same side. It is night and they have only one flashlight with them.
At most two men can cross the bridge at a time and any party who crosses, either one or two people, must have the flashlight with them.
The flashlight must be walked back and forth: it cannot be thrown, etc.
Each man walks at a different speed. A pair must walk together at the speed of the slower man.
Man 1 needs 1 minute to cross the bridge, man 2 needs 2 minutes, man 3 needs 5 minutes, and man 4 needs 10 minutes.
For example, if man 1 and man 3 walk across together, they need 5 minutes.

The question:
How can all four men cross the bridge in 17 minutes?
Table for Two ★★★

You are sitting with one opponent at an empty, round table.
Taking turns, you should place one euro on the table, in such a way that it touches none of the coins that are already on the table.
The first player that is not able to place a euro on the table has lost.
By tossing a coin, it has been decided that you may start.

The question:
Which strategy will you follow to make sure you are guaranteed to win?
To Know or not To Know ★★★

Two whole numbers, m and n, have been chosen.
Both are greater than 1 and the sum of them is less than 100.
The product, m × n, is given to mathematician X.
The sum, m + n, is given to mathematician Y.
Then both mathematicians have the following conversation:
X: "I have no idea what your sum is, Y."
Y: "That's no news to me, X. I already knew you didn't know that."
X: "Aha! Now I know what your sum must be, Y!"
Y: "And now I also know what your product is, X!"

The question:
What are the numbers m and n?

Another question:
Thanks to Yiheng Wang, we can present you the following puzzle:
There is a professor with three of her equally highly intelligent students (Amy, Brad, and Charles) and they are playing a puzzle game.
The professor puts a piece of paper on each student's forehead, and on each piece of paper, there is a positive integer number.
Each student can see the numbers on the other two students' foreheads, but not the one on him/herself.
The professor tells the students: out of these three positive integer numbers, one number equals to the sum of the other two.
The students cannot speak until the professor starts to ask question and the three students answer in order.
Professor: "Do you know the number on your forehead (for sure, no guessing)?"
Amy: "I don't know."
Brad: "I don't know."
Charles: "I don't know."
Then the professor starts the second round of questioning.
Professor: "Do you know the number on your forehead (for sure, no guessing)?"
Amy: "I don't know."
Brad: "I don't know."
Charles: "Yes. It's 144."
Question to you, the reader: what are the three numbers?
A Quiz ★★★
You are a participant in a quiz. The quizmaster shows you three closed doors.
He tells you that behind one of these doors, there is a prize, and behind the other two doors, there is nothing.
You select one of the doors,
but before you open it, the quizmaster deliberately picks out a remaining empty door and shows that there is nothing behind it.
The quizmaster offers you a chance to switch doors with the remaining closed door.

The question:
Should you stick to your choice?
Coin Weighing ★★★
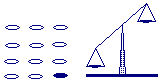
We have 12 coins and a balance. 11 coins are of the same weight, but one coin differs in weight
(note that you do not know whether the coin with different weight is heavier or lighter!).
You may perform three weighings to find out which coin has a different weight,
and whether this coin is heavier or lighter.

The question:
How should you perform these three weighings to find out which coin has a different weight,
and whether this coin is heavier or lighter?
The Masters Plaza ★★★
Thanks to Hassan Issa from Lebanon, we can present you the following puzzle:
You have the chance to take your room in the "Masters Plaza",
a hotel in which five masters (five of the most intelligent people who ever lived) are present.
The hotel consists of five rooms and a small restaurant that contains five tables.
Each master has a rank, which shows his level of thinking with respect to the whole group.
The master with the first rank is said to be the head master, and he is not you.
Rooms, as well as tables,
are successively numbered from 1 to 5 in a way that each master lives in a room and eats on a table different in number from his rank.
To avoid confrontation,
masters with successive ranks are allowed neither to live in rooms next to each other nor to eat on tables next to each other.
The four present masters are Albert Einstein, Galileo Galilei, Hassan Issa, and Archimedes.
To have your room in the Plaza, you just must know your rank, table number and room number knowing that:
- Archimedes does not eat on the fifth table.
- Einstein is not the head master.
- Archimedes has exactly the middle rank between Hassan and you.
- Einstein is more intelligent than Archimedes is.
- Galileo eats on a table next to that of Einstein.
- Hassan does not eat on a table with the same number as his room number.

The question:
What are the ranks, room numbers, and table numbers of the five masters?

The answer:
Click here!
Copyright © 2005 by Hassan Issa, Lebanon. Published on this website with permission.
3 Heads & 5 Hats ★★★

In a small village in the middle of nowhere, three innocent prisoners are sitting in a jail.
One day, the cruel jailer takes them out and places them in a line on three chairs,
in such a way that man C can see both man A and man B, man B can see only man A,
and man A can see none of the other men.
The jailer shows them 5 hats, 2 of which are black and 3 of which are white.
After this, he blindfolds the men, places one hat on each of their heads,
and removes the blindfolds again.
The jailer tells his three prisoners that if one of them can determine the color of his hat within one minute,
all of them are released. Otherwise, they will all be executed.
None of the prisoners can see his hat, and all are intelligent.
After 59 seconds, man A shouts out the (correct) color of his hat!

The question:
What is the color of man A's hat, and how does he know?
Zebra ★★★

There are five adjacent houses.
Each house has a unique color, and each owner has a different nationality.
Each owner keeps a different pet, drinks a different type of beverage, and has a different occupation.
The Brit lives in the red house, the Swede keeps a dog, and the Dane drinks tea.
The green house is on the immediate left of the white house.
In the green house, they drink coffee.
The postman has birds.
The fireman lives in the yellow house.
In the middle house, they drink milk.
The Norwegian lives in the leftmost house.
The baker lives in the house next to the house with the cats.
The fireman lives in the house next to the house with the horse.
The bus driver drinks lemonade.
The German is plumber.
The Norwegian lives next to the blue house.
They drink water in the house that lies next to the house where the baker lives.
One of the owners keeps a zebra.

The question:
Who owns the zebra?
Cube Creatures ★★★★
On the planet Gnirica grows the peculiar cube plant (cubus vulgaris gniricae);
see the image below.
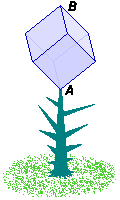
On the perfect cubical flower of this plant, the cube creature (ambulator cubi gniricae) lives.
This creature is born at the bottom of the flower (point A), and his whole life it walks along the sides of the cubical flower.
The cube creature walks one whole side in one year, and if it arrives in a vertex,
it chooses a new direction (it can also decide to walk back along the same side it came from).
As soon as the cube creature arrives at the top of the cubical flower (point B), it dies.
Consequently, cube creatures live at least three years.

The question:
What age do cube creatures reach on average?
Colorful Dwarfs ★★★★

In a distant, dark forest, lives a population of highly intelligent dwarfs.
The dwarfs all look exactly alike, but only differ in the fact that they are wearing either a red or a blue hat.
Striking however, is that none of the dwarfs knows what the color of his or her hat is.
This is because it is forbidden to speak about the color of the hats, and there no mirrors in the forest.
Nevertheless, the dwarfs do know that there is at least one dwarf with a red hat.
Once every century, there is a big party in this village, to which initially all dwarfs will go.
However, this party is only intended for dwarfs wearing a blue hat.
Dwarfs with a red hat are supposed not to return to the party the next day, as soon as they know that they are wearing a red hat.

The question:
How many days did it take before there were no more dwarfs with a red hat left at the last party,
if you know that there were then 250 dwarfs with a red hat and 150 dwarfs with a blue hat?

Another question:
The dwarfs prefer a faster way to separate the red hats from the blue hats, how can they accomplish this?
Pirate Treasure ★★★★

A pirate ship captures a treasure of 1000 golden coins.
The treasure must be split among the five pirates: 1, 2, 3, 4, and 5 in order of rank.
The pirates have the following important characteristics:
- Infinitely smart.
- Bloodthirsty.
- Greedy.
Starting with pirate 5, they can make a proposal how to split up the treasure.
This proposal can either be accepted or the pirate is thrown overboard.
A proposal is accepted if and only if the majority of the pirates agrees on it.

The question:
What proposal should pirate 5 make?
Bizarre Boxes ★★★★

Someone shows you two boxes and he tells you that one of these boxes contains two times as much as the other one,
but he does not tell you which one this is.
He lets you choose one of these boxes and opens it.
It turns out to be filled with $10.
Now he gives you the opportunity to choose the other box instead of the current one (and skip the $10 of the first box),
because the second box could contain twice as much (i.e. $20).

The question:
Should you choose the second box, or should you stick to your first choice to maximize the expected amount of money?

A hint :
If you have $10, and you could double this with a chance of 1/2, or half it with a chance of 1/2,
one would expect an average of 1/2 * $20 + 1/2 * $5 = $12.5 (so you would expect to gain $2.5)!
The Truel ★★★★

On an early morning, three rivals get together on an open spot in a dark wood to compose a quarrel by means of guns.
A kind of duel, but with three persons: A, B, and C.
The rules of the game are:
- they draw lots who may fire first, second and third;
- next, they will continue firing at each other in this order until only a single person is alive;
- every person decides himself at which person he fires;
- everyone knows that A hits (kills) in 100% of all shots, B hits (kills) in 80% of all shots and C hits (kills) in 50% of all shots;
- each person chooses his ideal strategy;
- no one is killed by a stray bullet.

The question:
Who has the largest chance of surviving the truel,
and how big is this chance?
Fourteen Fifteen ★★★★
Below you see a square with fifteen numbered, movable tiles and an empty space in the lower right corner.
The tiles are placed in the correct order, except for the tiles numbered "14" and "15", which have been swapped.

The question:
How must the tiles be moved, to get all tiles in the correct order,
with the empty space again in the lower right corner?

A hint :
Clicking the mouse on a tile next to the empty space moves the tile into the empty space.
By clicking the mouse on a tile in the same row or column as the empty space, you can move several tiles in one move.

Another question:
Starting from the begin position, how must the tiles be moved, to get all tiles in the correct order,
but with the empty space in the upper left corner (see below)?
Coconut Chaos ★★★★

Five sailors survive a shipwreck and swim to a tiny island where there is nothing but a coconut tree and a monkey.
The sailors gather all the coconuts and put them in a big pile under the tree.
Exhausted, they agree to go to wait until the next morning to divide the coconuts.
At one o'clock in the morning, the first sailor wakes up.
He realizes that he cannot trust the others and decides to take his share now.
He divides the coconuts into five equal piles, but there is one coconut left over.
He gives that coconut to the monkey, hides his coconuts (one of the five piles) and puts the rest of the coconuts
(the other four piles) back under the tree.
At two o'clock, the second sailor wakes up.
Not realizing that the first sailor has already taken his share,
he too divides the coconuts up into five piles,
leaving one coconut over which he gives to the monkey.
He then hides his share (one of the five piles),
and puts the remainder (the other four piles) back under the tree.
At three, four, and five o'clock in the morning,
the third, fourth, and fifth sailors each wake up and carry out the same actions.
In the morning, all the sailors wake up, and try to look innocent.
No one makes a remark about the diminished pile of coconuts,
and no one decides to be honest and admit that they have already taken their share.
Instead, they divide the pile up into five piles, for the sixth time,
and find that there is yet again one coconut left over, which they give to the monkey.

The question:
What is the smallest amount of coconuts that there could have been in the original pile?
Numbers and Dots ★★★★

This is a famous problem from 1882, to which a prize of $1000 was awarded for the best solution.
The task is to arrange the seven numbers 4, 5, 6, 7, 8, 9, and 0,
and eight dots in such a way that an addition approximates the number 82 as close as possible.
Each of the numbers can be used only once. The dots can be used in two ways: as decimal point and as symbol for a recurring decimal.
For example, the fraction 1/3 can be written as
The dot on top of the three denotes that this number is repeated infinitely.
If a group of numbers needs to be repeated, two dots are used:
one to denote the beginning of the recurring part and one to denote the end of it.
For example, the fraction 1/7 can be written as
Note that '0.5' is written as '.5'.

The question:
How close can you get to the number 82?